Getting to correlation

While Test & Measurement companies strive to ensure their equipment makes accurate measurements that agree from instrument to instrument, discrepancies do arise. Making the same measurement on two different measurement platforms can generate disparate results that demand explanation and/or correction. These issues are often exacerbated when making the same measurements on test equipment with inherently different architectures. Oscilloscope users must consider that real-time and sampling oscilloscopes have fundamental differences in how they sample and analyse waveform data when setting up measurements and interpreting results. For jitter measurements in particular, separating jitter into components down to low Bit Error Ratios (BER) on an oscilloscope is not a direct measurement, rather it is an extrapolation from the acquired data that in itself can introduce some error.

In the past, these discrepancies between sampling and real-time scopes were mostly negligible as slower data rates meant greater design margins. For instance, a 1Gbit/s signal has a unit interval of 1000ps. An extra 10ps of jitter on your measurement represents only 1% of the unit interval. This is unlikely to cause compliance failures or raise any red flags. At 28Gbit/s the unit interval has closed to 36ps, firmly in the regime of fighting for every last picosecond of margin. Measurement discrepancies between instruments can easily produce the inconclusive results and the accompanying uncertainty and frustration. Despite these challenges, it is possible to consistently achieve good jitter measurement correlation between real-time and sampling oscilloscopes.

Oscilloscope architectures

While both are oscilloscopes, and thus able to display and analyse waveforms versus time, there are fundamental differences in the way real-time scopes and sampling scopes sample and reconstruct the incoming signal. These differences play an important role in understanding the strengths and limitations of each instrument as well as measurement discrepancies between them. Figure 1 shows simplified block diagrams of a real-time and sampling oscilloscope.

Figure 1: Over-simplified block diagram of real-time oscilloscope (top) and sampling oscilloscope (bottom).

There are a few critical differences in these architectures to point out. A real-time scope must sample the waveform fast enough to faithfully reconstruct it after a single shot in real time. This means the sample rates of the ADC must be faster than twice the highest frequency component of the signal, thereby obeying Shannon-Nyquist sampling theory. With real-time oscilloscopes now exceeding 60GHz of bandwidth, this requires extreme ADC sample rates. At the time of this writing the fastest such ADCs provide 160GSa/s in a single real-time scope channel. Such a fast sample rate allows the real-time scope to be an extremely powerful and flexible general purpose instrument. The scope can capture single shot, low probability of occurrence waveforms that a sampling scope cannot reliably measure.

A sampling oscilloscope, on the other hand, samples the waveform in ‘equivalent time.’ The scope will analyse many passes of the input signal, taking one sample during each pass. The acquisition processor triggers the sampler to output a very short pulse to sample the waveform. This sample is then passed through an amplifier to scale it over the input range of the ADC before being digitised. By taking many such samples with very accurate timing resolution the waveform can be accurately reconstructed. Critically, bandwidth is determined by the width of the sample pulse and the timing accuracy of the sample, not the sample rate of the ADC. Sampling scopes with bandwidths as high as 90GHz use ADCs that sample at tens to hundreds of KSa/s. This, combined with the elimination of the attenuator on the front end of a real-time scope, generally allows a sampling scope to sample with lower noise and greater vertical resolution.

A sampling scope requires an external clock or separate hardware clock recovery circuitry. In contrast, a real-time scope samples fast enough to recover a clock in software from the digitised waveform data. This provides greater flexibility in clock recovery settings when making jitter measurements. Furthermore, the real-time scope architecture allows for trigger control circuitry directly off the pre-amp. This allows for extremely robust and flexible triggering options to ensure the user is able to trigger on precisely the signal behaviour desired. By nature of having to build up the reconstructed waveform more slowly, a sampling scope provides relatively basic triggering options.

Clock recovery

Jitter must be measured versus a reference clock. A discrete clock can be provided as reference, or, in many cases, the reference clock is recovered from the transmitted data signal. When setting up a jitter measurement, it is often desirable for the oscilloscope to emulate the clock recovery behaviour of the data receiver to isolate the data transmitter and channel during testing. To this end, many high speed digital standards specify the clock recovery settings to be used when testing devices. Among the most common clock recovery methods is the phase locked loop (PLL). There are important nuances when setting up PLL clock recovery that can have a dramatic impact on jitter measurement results; order, loop bandwidth, and damping factor.

Figure 2: Plot of phase locked loop response versus frequency. Jitter transferred from signal to recovered clock shown in blue (JTF). Jitter transferred from signal to the observed measurement shown in pink (OJTF).

The ideal, jitter-free reference clock does not exist. When a PLL is used to recover a clock, some of the jitter on the original data signal is transferred to the clock signal. An illustrative way to view this jitter transfer is plotting the jitter transfer function (JTF) and observed jitter transfer function (OJTF) versus frequency (Figure 2). The PLL can be thought of as a low pass filter that gates what frequencies of jitter on the data signal are transferred to the reference clock. The low pass filter response can be seen in the JTF in Figure 2. The order of the PLL determines the roll-off behaviour of the filter (20db/dec for 1st order, 40db/dec for 2nd order, etc.). The loop bandwidth is the 3dB point in the filter roll-off. The damping factor determines how much peaking occurs in the filter response. For a PLL with 10 MHz loop bandwidth, it is a reasonable approximation to assume jitter below 10MHz will be transferred to the reference clock while jitter above 10MHz will be filtered out by the PLL. Further, significant peaking in the JTF can cause the jitter band that aligns with the peaked response to be amplified on the reference clock.

How does this affect the jitter measurement on the data signal? Since jitter is measured relative to the recovered reference clock, any jitter that is transferred to the reference clock will be ‘tracked out’ of the resulting jitter measurement. This gives rise to the observed jitter transfer function (OJTF), the pink trace shown in Figure 2. The OJTF is a high pass filter that indicates what jitter, present on the data signal, will be observed by the receiver or oscilloscope.

When setting up a jitter measurement comparison on a sampling scope and a real-time scope it is critical that the same PLL order, loop bandwidth and damping factor be used for both platforms to achieve correlation. The way these PLL settings are specified can make this challenging, in particular regarding the loop bandwidth. Some high speed digital standards specify the JTF loop bandwidth and some specify the OJTF loop bandwidth. Further, oscilloscopes also differ on the default loop bandwidth specified for clock recovery. While the loop bandwidths of the JTF and OJTF response functions are the same for first order PLLs, this is not the case of PLLs of higher order, including the most commonly used 2nd order loop. In fact, for a 2nd order PLL the approximate rule of thumb is the JTF loop bandwidth is equal to two times the OJTF loop bandwidth. This difference in how much low frequency jitter is tracked out of the measurement, which can have dramatic results on jitter measurement correlation. The user must be very careful to know which loop bandwidth their standard is specifying and which their oscilloscope is setting.

Noise, edge speed and bandwidth

Figure 3: Accurate jitter measurements require oscilloscopes with the lowest noise and jitter measurement floor. Shown are the Agilent 86100D sampling oscilloscope (left) and Agilent 90000 Q-Series real-time oscilloscope.

In any measurement the oscilloscope itself will contribute some noise and jitter to the signal. Naturally, this can have a significant effect on jitter measurement results. Further, differences between instruments in how much noise and jitter they contribute can produce measurements that do not correlate. There is an inherent relationship between the noise floor of the oscilloscope, the bandwidth of the measurement, the edge speeds of the signal under test, and the jitter the scope contributes to the measurement. As a scope captures a waveform, the noise floor is superimposed on the measured signal. During a bit transition the vertical noise on the edge not only produces a vertical displacement of the waveform, but also a horizontal displacement that contributes to timing jitter. The faster the edge speeds of the signal, the less vertical noise will translate into horizontal jitter. Slower edge speeds are far more susceptible to this ‘AM to PM’ conversion. Thus, it is important to measure data signals with sufficient bandwidth to ensure the oscilloscope is not slowing measured edge speeds. This bandwidth requirement is often more important than the traditional view of scope bandwidth enabling capture of higher order harmonics. On the other hand, measuring signals with more scope bandwidth increases the broadband AC RMS noise the scope contributes to the signal. Therefore, the best practice is to use oscilloscopes that offer the lowest available noise floor and jitter measurement floor (Figure 3) and measure signals with just enough bandwidth to ensure edge speeds are accurately captured.

How oscilloscopes handle crosstalk-induced jitter can also produce dramatic disparities in jitter measurements. A detailed discussion of the taxonomy of jitter and the measurement thereof would require many dedicated articles on its own. For the purposes of this discussion, the traditional ‘spectral algorithm’ by which most oscilloscopes extract the random component of jitter significantly over-reports random jitter (RJ) for signals subject to crosstalk. Not only does this result in an erroneously high measurement of random jitter, but when combining jitter components to calculate total jitter (TJ) one of the terms is 14*RJ (for a Bit Error Ratio of 10^-12). Thus, any error in RJ produces a 14 times greater error in TJ.

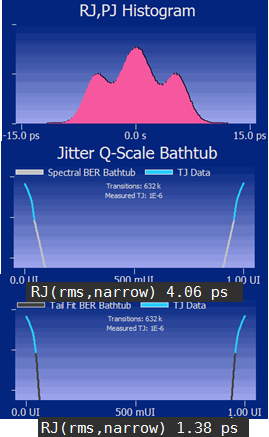
Figure 4: Using Agilent’s N5400A EZJIT+ software to compare jitter measurements on a signal with significant crosstalk using different RJ extraction algorithms. RJ/PJ histogram is shown at the top. The middle plot shows the BER bathtub curve and RJ RMS (4.06ps) value using a spectral algorithm for RJ extraction. The bottom plot shows the BER Bathtub curve and RJ RMS (1.38ps) value using a tail-fit algorithm for RJ extraction. Notice the improvement in bathtub curve shape and reduction in RJ RMS when using the tail-fit algorithm.

Some scopes now offer a second algorithm that performs a tail-fit to the jitter histogram to uniquely extract RJ before separating the component of jitter due to crosstalk. In fact, some standards, such as Thunderbolt now require crosstalk measurements using these tail fit algorithms as part of standard compliance testing. Of course correlation issues can arise. Comparing a spectral and tail fit extraction can generate very different results, as shown in Figure 4. Further, these tail-fit algorithms can be sensitive to the amount of data the scope acquires and different implementations can produce discrepancies. When comparing jitter measurements between oscilloscopes, be sure to account for the RJ extraction algorithm being used and any sources of crosstalk that may be present.

While tools exist that make jitter measurement setup on an oscilloscope fast and easy, there are many important details that can have a significant effect on the results of the measurement. Often these details become most apparent when comparing jitter results across oscilloscope platforms. Settings such as clock recovery, scope bandwidth, and random jitter extraction algorithm can cause divergent jitter measurements on the same data signal. The oscilloscope user must take care to ensure a truly ‘apples-to-apples’ measurement is being made and account for the intrinsic noise and jitter differences between the instruments to achieve confident jitter measurement correlation.